Astronomy and Speculation into the Celestial Harmonies

Book V

Ch. III

Summary of Astronomical Theory, Necessary for the Study of the Heavenly Harmonies.

To start with, readers should know that the ancient astronomical hypotheses of Ptolemy, in the way in which they have been expounded in the Theoricae of Peurbach and in the other writers of Epitomes, have been totally excluded from this discussion, and put out of mind; for they do not convey truthfully either the arrangement of the bodies in the world or the commonwealth of the motions.

In their place I cannot do other than substitute solely the opinion of Copernicus on the world, and, if it were possible, persuade everyone to believe it. Yet it is still a new idea to the common herd of scholars, and a doctrine which to many is quite absurd to hear, that the Earth is one of the planets, and is carried among the stars round an unmoving Sun. Therefore, those who are shocked at the novelty of this opinion should know that these speculations about harmonies also find a place in the hypotheses of Tycho Brahe, because that author has everything else which relates to the arrangements of the bodies and the combination of their motions in common with Copernicus. It is only the Copernican annual motion of the Earth which he transfers to the whole system of the planetary spheres, and to the Sun, which occupies the middle by the agreement of both authors. For by this transference of the motion it nevertheless comes about that the Earth, if not in that vast and immense space within the sphere of the fixed stars, yet at least in the system of the planetary world, takes the same position at any given time according to Brahe as Copernicus gives it. In fact, just as someone who draws a circle on paper moves the writing leg of his compasses round, whereas someone who fastens his paper or tablet to a revolving wheel describes the same circle, without moving the leg of his compasses or his pen, on the tablet as it moves round; in the same way in this case for Copernicus indeed the Earth traces out a circle by a real motion of its own body, passing in between the circles of Mars on the outside and Venus on the inside; but for Tycho Brahe the whole planetary system (in which among the others are also the circles of Mars and Venus) turns, like the tablet on the wheel, applying to the motionless Earth, as if to the pen of the man who turns the wheel, the blank space between the circles of Mars and Venus. The effect of this motion of the system is that the Earth marks on it the same circle round the Sun, intermediate between those of Mars and Venus, while itself it remains motionless, as according to Copernicus it marks out by a true motion of its own body, with the system at rest. Therefore, as harmonic study considers the motions of the planets as eccentric, as if viewed from the Sun, it may readily be understood that if an observer were on the Sun, even though it were in motion, to him the Earth, although it were at rest (to make a concession already to Brahe), would nevertheless appear to be going around an annual circuit, placed in between the planets, and also in an intermediate period of time. Hence if there is a man whose confidence is too weak for him to be able to accept the motion of the Earth among the stars, nevertheless he will be able to rejoice in the marvellous study of this absolutely divine mechanism, if he applies whatever he is told about the daily motions of the Earth on its eccentric to their appearance from the Sun, the same appearance as Tycho Brahe shows, with the Earth at rest.

However, true enthusiasts for the Samian philosophy have no just cause to grudge such people this share in a most delightful speculation, inasmuch as their joy will be many times more perfect, that is from the complete perfection of the speculation, if they do also accept the immobility of the Sun and furthermore the movement of the Earth.

First, therefore, readers should take it as absolutely settled today among all astronomers that all the planets go round the Sun, with the exception of the moon, which alone has the Earth as its center; and its orbit or course is not large enough to be capable of being drawn in its correct proportion to the rest in the following plan. Therefore the Earth is added to the other five as a sixth, which either by its own motion, with the Sun at rest, or without moving itself while the whole system of the planets revolves, itself also marks out a sixth circle round the Sun.

Second, it is also settled that all the planets are eccentric, that is, they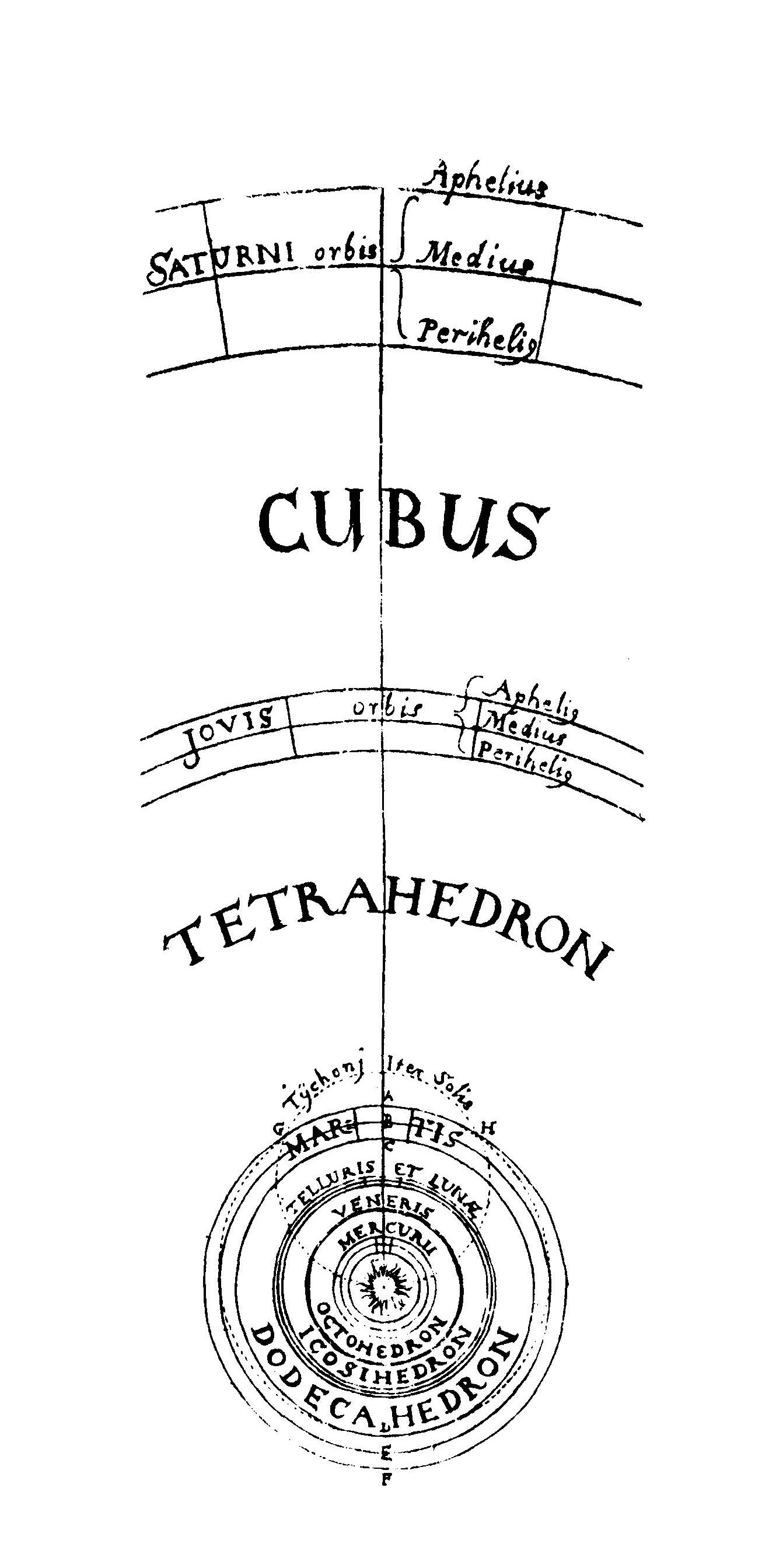 change their distances from the Sun, in such a way that on one side their orbits are furthest from the Sun, in the other they come closest to the Sun.

[...]

Third, the reader should remember what I published in The Secret of the Universe, 22 years ago, that the number of the planets, or of courses round the Sun, was taken by the most wise Creator from the five regular solid figures, about which Euclid so many centuries ago wrote a book which is called the Elements, on account of its being made up of a series of Propositions. However, the fact that there cannot be more regular solids, that is, that regular plane figures cannot be congruent in a solid in more than five ways, has been made clear in Book II of this work.

Fourth, as far as the proportion of the planetary orbits is concerned, between pairs of neighboring orbits indeed it is always such as to make it readily apparent that in each case the proportion is close to the unique proportion of the spheres of one of the solid figures, that is to say the proportion of the circumscribed sphere of the figures to the inscribed sphere.

[...]

From that fact it is evident that the actual proportions of the planetary distances from the Sun have not been taken from the regular figures alone; for the Creator, the actual fount of geometry, who, as Plato wrote, practices eternal geometry, does not stray from his own archetype. And that could certainly be inferred from the very fact that all the planets change their intervals over definite periods of time, in such a way that each one of them has two distinctive distances from the Sun, its greatest and its least; and comparison of distances from the Sun between pairs of planets is possible in four ways, either of the greatest distances, or of the least, or of the distances on opposite sides when they are furthest from each other, or when they are closest. Thus the comparisons between pair and pair of neighboring planets are twenty in number, whereas on the other hand there are only five solid figures. However, it is fitting that the Creator, if He paid attention to the proportion of the orbits in general, also paid attention to the proportion between the varying distances of the individual orbits in particular, and that that attention should be the same in each case, and that one should be linked with another. On careful consideration, we shall plainly reach the following conclusion, that for establishing both the diameters and the eccentricities of the orbits in conjunction, more basic principles are needed in addition to the five regular solids.

Fifth, to come to the motions, between which harmonies are established, I again impress on the reader that it was shown by me in my Commentaries on Mars, from the thoroughly reliable observations of Brahe, that equal daily arcs on one and the same eccentric are not traversed at the same speed;

8. and in consequence, since the proportion of the circles is the same as that of their diameters, that the proportion of one mean daily arc to the sum of all the mean daily arcs making up the whole circuit, which are equal to each other, is the same as that of a mean daily arc to the sum of all the true eccentric arcs, which are the same in number but unequal to each other. We need to have this knowledge of the true eccentric daily arcs, and of the true . motions, beforehand so that we can now grasp through them the apparent motions, supposing the eye to be at the Sun.

Sixth, as far indeed as concerns the apparent arcs as seen from the Sun, it has been known even from the time of ancient astronomy that of the true motions, even those which are equal to each other, one which has moved further away from the center of the world (such as one which is at aphelion) seems to be smaller, to an observer at that center; and one which is nearer, such as one which is at perihelion, also seems to be greater.

[...]

Eighth, up till now we have dealt with the various elapsed times or arcs of one and the same planet. Now we must also deal with the motions of pairs of planets compared with each other. Here note the definitions of the terms which we are going to need. We shall call the nearest apsides of two planets the perihelion of the upper one and the aphelion of the lower one, notwithstanding the fact that they are tending not to the same side of the world, but to different, and perhaps opposite sides.

2. By extreme motions understand the slowest and the fastest of the whole planetary circuit.

3. By converging or approaching motions, those which are at the nearest apsides of the two, that is at the perihelion of the upper planet and the aphelion of the lower;

4. by diverging or receding motions, those which are at opposite apsides, that is at the aphelion of the upper planet and the perihelion of the lower. Again, therefore, a part of my Secret of the Universe, put in suspense 22 years ago because it was not yet clear, is to be completed here, and brought in at this point. For when the true distances between the spheres were found, through the observations of Brahe, by continuous toil for a very long time, at last, at last, the genuine proportion of the periodic times to the proportion of the spheres —

only at long last did she look back at him as he lay motionless. But she looked back and after a long time she came

and if you want the exact moment in time, it was conceived mentally on the 8th March in this year one thousand six hundred and eighteen, but submitted to calculation in an unlucky way, and therefore rejected as false, and finally returning on the 15th of May and adopting a new line of attack, stormed the darkness of my mind. So strong was the support from the combination of my labor of seventeen years on the observations of Brahe and the present study, which conspired together, that at first I believed I was dreaming, and assuming my conclusion among my basic premises. But it is absolutely certain and exact that the proportion between the periodic times of any two planets is precisely the sesquialterate proportion of their mean distances, that is, of the actual spheres, though with this in mind, that the arithmetic mean between the two diameters of the elliptical orbit is a little less than the longer diameter. Thus if one takes one third of the proportion from the period, for example, of the Earth, which is one year, and the same from the period of Saturn, thirty years, that is, the cube roots, and one doubles that proportion, by squaring the roots, he has in the resulting numbers the exactly correct proportion of the mean distances of the Earth and Saturn from the Sun. For the cube root of 1 is 1, and the square of that is 1. Also the cube root of 30 is greater than 3, and therefore the square of that is greater than 9. And Saturn at its average distance from the Sun is a little higher than nine times the average distance of the Earth from the Sun. The use of this theorem will be necessary in Chapter IX for the derivation of the eccentricities.